It is not always possible to determine the existence of a limit cycle directly from the polar form as is illustrated in the following example.

• Worked Example 1

has a stable limit cycle which lies in the annular region

The polar form of the system is

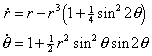
Note that the polar form does not immediately give a limit cycle.

If 

But this must be true for all . This means that

If 

But this must be true for all . This means that

These results imply that when and when .

Consider a trajectory which starts inside the annular region shown below.

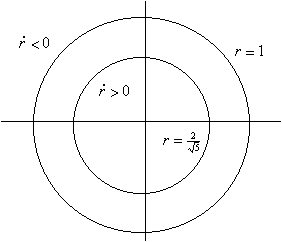
Suppose that the trajectory touches the outer boundary. Then immediately and the trajectory is forced to remain in the annulus. Similarly if the trajectory touches the inner boundary then and once more the trajectory is forced to remain in the annulus. Thus any trajectory which starts inside the annulus is trapped within it. Such a region is called a trapping region.

There are no equilibrium points within the annulus. Intuitively since a trajectory cannot tend to an equilibrium point and cannot cross itself the trajectory must either be a closed orbit or eventually tend to a closed orbit. Hence there must be at least one limit cycle within the annulus.